Matrice de rotation
Soit la matrice de rotation suivante : $$[ x_2 y_2 z_2]=\begin{pmatrix} x_1x_2 & x_1y_2 & x_1z_2 \\ y_1x_2 & y_1y_2 & y_1z_2 \\ z_1x_2 & z_1y_2 & z_1z_2 \\\end{pmatrix}$$
Pour la rotation autour de zLe vecteur $z$ étant vers nous, l'angle $\alpha$ est donc positif.
On peut donc dire : $x_1x_2$ est la projection de $x_2$ sur $x_1$ soit : $x_1x_2=cos \alpha$
| $x_1x_2=cos \alpha$ | $x_1y_2=-sin \alpha$ | $x_1z_2=0$ |
| $y_1y_2=cos \alpha$ | $y_1y_2=sin \alpha$ | $y_1z_2=0$ |
| $z_1x_2=0$ | $z_1y_2=0$ | $z_1z_2=1$ |
Le vecteur $y$ étant vers l'arrière, l'angle $\beta$ est donc négatif.
On peut donc dire : $x_1x_2$ est la projection de $x_2$ sur $x_1$ soit : $x_1x_2=cos -\beta=cos \beta$
| $x_1x_2=cos -\beta=cos \beta$ | $x_1y_2=0$ | $x_1z_2=-sin -\beta=sin \beta$ |
| $y_1x_2=0$ | $y_1y_2=1$ | $y_1z_2=0$ |
| $z_1x_2=sin -\beta=-sin \beta$ | $z_1y_2=0$ | $z_1z_2=cos -\beta=cos \beta$ |
Le vecteur $x$ étant vers nous, l'angle $\gamma$ est donc positif.
On peut donc dire : $y_1y_2$ est la projection de $y_2$ sur $y_1$ soit : $y_1y_2=cos \gamma$
| $x_1x_2=1$ | $x_1y_2=0$ | $x_1z_2=0$ |
| $y_1y_2=0$ | $y_1y_2=cos \gamma$ | $y_1z_2=-sin \gamma$ |
| $z_1x_2=0$ | $z_1y_2=sin \gamma$ | $z_1z_2=cos \gamma$ |
Soit un vecteur $x_1\begin{vmatrix} x \\ y \\ z \\ \end{vmatrix}$
et le vecteur tourner autour de z $x_2\begin{vmatrix} x' \\ y' \\ z' \\ \end{vmatrix}$
Pour la rotation nous allons donc effectuer l'opération $x_2=Rot_Z \times x_1$ soit $$x_2=\begin{pmatrix} cos \alpha & -sin \alpha & 0 \\ sin \alpha & cos \alpha & 0 \\ 0 & 0 & 1 \\\end{pmatrix} \times \begin{pmatrix} x \\ y \\ z \\ \end{pmatrix}=\begin{pmatrix} x\cdot cos \alpha-y\cdot sin \alpha \\ x\cdot sin \alpha+y\cdot cos \alpha \\ z \\ \end{pmatrix}$$
Exemple d'application

Soit une toiture dont une pente fait un angle de 47° dans le plan yz et une pente de 50° dans le plan xy. On souhaite donnée l'équation de l'arêtier afin de connaitre les angles de coupe des ardoise ainsi que le nombre de biaises. Rappel du produit vectoriel symbole $\otimes$ ou $\wedge$
On effectue tout d'abord une rotation d'une droite le long de x de longueur unitaire autour de z
$\vec u=\begin{vmatrix} 1 \\ 0 \\ 0 \\ \end{vmatrix}$
$\vec {u'}=\begin{pmatrix} cos 50° & -sin 50° & 0 \\ sin 50° & cos 50° & 0 \\ 0 & 0 & 1 \\\end{pmatrix} \times \begin{vmatrix} 1 \\ 0 \\ 0 \\ \end{vmatrix}=\begin{vmatrix} cos 50° \\ sin 50° \\ 0 \\ \end{vmatrix}$
Puis son vecteur normal soit le produit vectoriel avec une droite le long de z de longueur unitaire
$\vec {u''}=\begin{vmatrix} cos 50° \\ sin 50° \\ 0 \\ \end{vmatrix} \wedge \begin{vmatrix} 0 \\ 0 \\ 1 \\ \end{vmatrix}=\begin{vmatrix} -sin 50° \\ cos 50° \\ 0 \\ \end{vmatrix}$
On effectue tout d'abord une rotation d'une droite de longueur unitaire le long de z autour de x de 47°
$\vec v=\begin{vmatrix} 0 \\ 0 \\ -1 \\ \end{vmatrix}$
$\vec {v'}=\begin{pmatrix} 1 & 0 & 0 \\ 0 & cos 47° & -sin 47° \\ 0 & sin 47° & cos 47° \\\end{pmatrix} \times \begin{vmatrix} 0 \\ 0 \\ -1 \\ \end{vmatrix}=\begin{vmatrix} 0 \\ sin 47° \\ -cos 47° \\ \end{vmatrix}$
Puis son vecteur normal soit le produit vectoriel avec une droite le long de x de longueur unitaire
$\vec {v''}=\begin{vmatrix} 0 \\ sin 47° \\ -cos 47° \\ \end{vmatrix} \wedge \begin{vmatrix} -1 \\ 0 \\ 0 \\ \end{vmatrix}=\begin{vmatrix} 0 \\ cos 47° \\ sin 47° \\ \end{vmatrix}$
Ensuite on calcul le produit vectoriel des deux vecteurs normaux (en effet, nous nous retrouvons avec le vecteur directeur de la droite d'intersection)
$\vec w=\vec {u''} \wedge \vec {v''}=\begin{vmatrix} -sin 50° \\ cos 50° \\ 0 \\ \end{vmatrix} \wedge \begin{vmatrix} 0 \\ cos 47° \\ sin 47° \\ \end{vmatrix}=\begin{vmatrix} cos 50° \cdot sin 47° \\ sin 50° \cdot sin 47° \\ -sin 50° \cdot cos 47° \\ \end{vmatrix}=\begin{vmatrix} 0,470105098 \\ 0,560249439 \\ -0,522441054 \\ \end{vmatrix}$
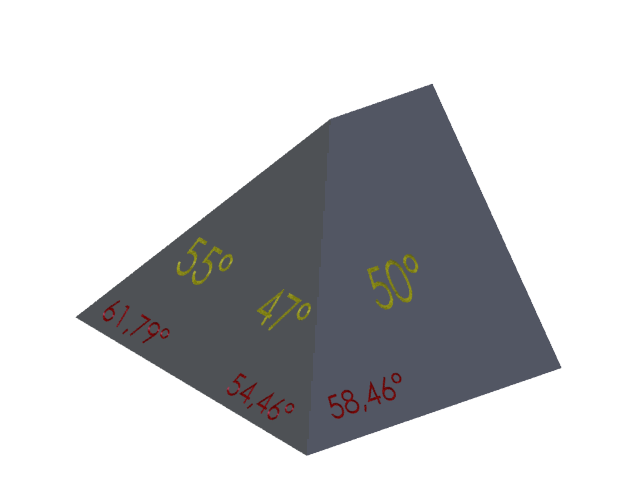
Et enfin l'angle entre $\vec w$ et $\vec x$
$\| \vec w \|=0,898789682$
$\vec w \cdot \vec x=0,470105098$
$\theta_1 = \arccos\left(\dfrac {\vec w \cdot \vec x}{\| \vec w\|\times \| \vec x \|}\right)$
Donc $\theta_1 = \arccos \dfrac {0,898789682}{0,470105098}=58,46344925°$
De la même manière (attention au signe) : $\theta_2 =\arccos\left(\dfrac {\vec w \cdot \vec {-z}}{\| \vec w\|\times \| \vec x \|}\right) \arccos \dfrac {0,898789682}{0,522441054}=54,45995922°$
si $\vec u\begin{vmatrix} x_1 \\ y_1 \\ z_1 \\ \end{vmatrix}$ et $\vec v\begin{vmatrix} x_2 \\ y_2 \\ z_2 \\ \end{vmatrix}$
$\vec u\otimes \vec v=\begin{vmatrix} y_1\cdot z_2 - y_2 \cdot z_1 \\ x_2\cdot z_1 - x_1 \cdot z_2 \\ x_1\cdot y_2 - x_2 \cdot y_1 \\ \end{vmatrix}$
Nous allons ensuite trouver les deux angles qui nous interresse grace au produit scalaire
Rappel du produit scalaire symbole $\cdot$
si $\vec u\begin{vmatrix} x_1 \\ y_1 \\ z_1 \\ \end{vmatrix}$ ; $\vec v\begin{vmatrix} x_2 \\ y_2 \\ z_2 \\ \end{vmatrix}$ ;
$\vec u\cdot \vec v=\begin{vmatrix} x_1\cdot x_2 \\ y_1 \cdot y_2 \\ z_1\cdot z_2 \\ \end{vmatrix}$
Mais également : $\theta = \arccos\left(\dfrac {\vec u \cdot \vec v}{\| \vec u\|\times \| \vec v \|}\right)$
$\| \vec u\|=\sqrt{x_1^2+y_1^2+z_1^2}$ ; $\| \vec v\|=\sqrt{x_2^2+y_2^2+z_2^2}$
Rappel du produit matrice x vecteur
soit un vecteur $\vec u=\begin{vmatrix} u_1 \\ u_2 \\ _u3 \\ \end{vmatrix}$
et une matrice $M=\begin{pmatrix} a_{11} & a_{12} & a_{13} \\ a_{21} & a_{22} & a_{23} \\ a_{31} & a_{32} & a_{33} \\\end{pmatrix}$
alors $\vec u \times M=\begin{pmatrix} a_{11}\cdot u_1 & a_{12}\cdot u_2 & a_{13}\cdot u_3 \\
a_{21}\cdot u_1 & a_{22}\cdot u_2 & a_{23}\cdot u_3 \\
a_{31}\cdot u_1 & a_{32}\cdot u_2 & a_{33}\cdot u_3 \\\end{pmatrix}$